
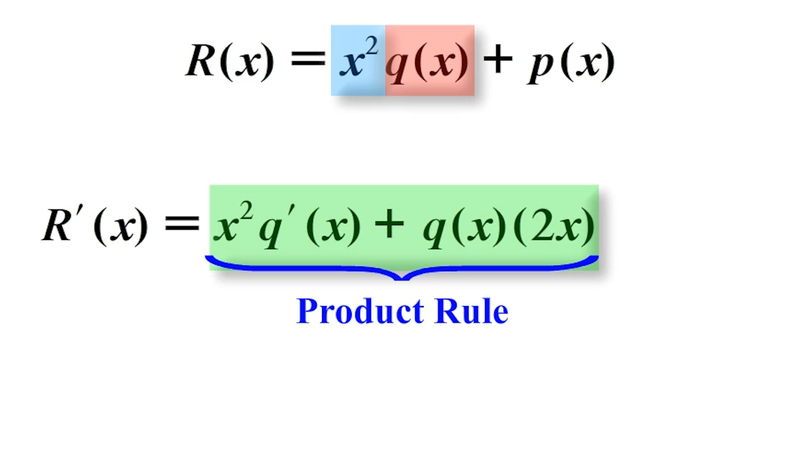
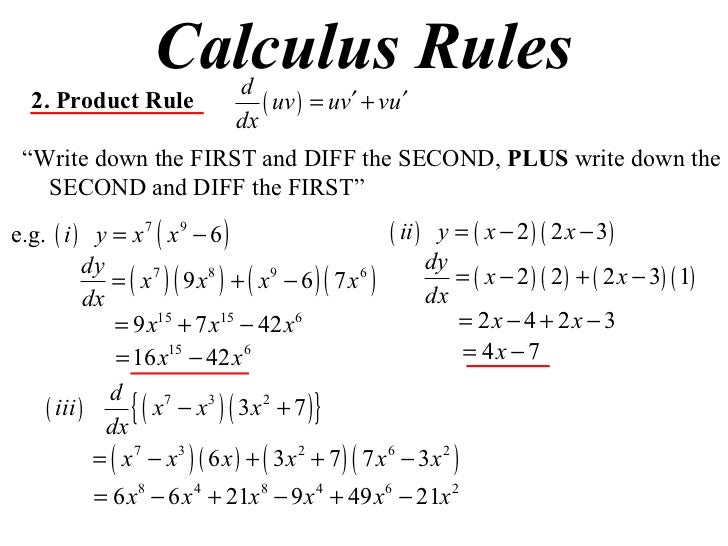
Write coherent and logical solutions to mathematical problems. Demonstrate ability to effectively interpret and use functions of several variables including finding limits, the use of partial derivatives, and finding and analyzing critical points of these functions with and without constraints. Understand the concepts of vectors, and vector valued functions in two and three dimensions. Understand lines, curves, planes, surfaces and graphs of functions in two variables in three dimensional coordinate systems Specific topics include: vectors, dot and cross products, equations of lines and planes, polar, cylindrical and spherical coordinates, differentiation of vector functions, velocity and acceleration, arc length, parametric surfaces, functions of several variables, partial derivatives, tangent plane and linear approximations, chain rule for partial derivatives, directional derivative and gradient, max-min problems for functions of several variables, Lagrange multipliers. it will develop techniques to obtain local linear approximations of functions (of multiple variables) in order to analyze and optimize quantities. This course will extend the methods of single-variable calculus to functions of many variables, i.e.

At UIC she has been a member of the Developmental Math Task Force, which is a part of the Student Success Initiative, as well as a Master Teaching Scholar for the second year of the Center for Teaching and Learning Communities. Outside of teaching she received the Best Practices University Excellence Award, and the Campus Partner Award (Student Affairs) at Northwestern. Awards during the 15-year career at Northwestern include 10 teaching awards, including the Northwestern WCAS Teaching Alumni award, the Northwestern Charles Deering McCormick University Distinguished Lecturer Award, and eight-time recipient of the ASG Honor Roll. Prior to coming to UIC and NU, she taught at Brandeis, Tufts, Wellesley and Harvard. Martina Bode was the director of calculus at Northwestern University for 15 years before accepting her position as director of calculus at UIC in the fall of 2015.
There is also an excellent Calc III review sheet made by a friend of mine from the University of Connecticut, which I have also included here. The content is based on MATH 13 at Tufts University and follows closely the text of Calculus – Early Transcendentals by Briggs and Cochran.Ĭhapter 11 – Vectors and Vector-Valued Functionsġ1.1 – Vectors in the Plane, 11.2 – Vectors in Three Dimensions, 11.3 – Dot Products, 11.4 – Cross Products, 11.5 – Lines and Curves in Space, 11.6 – Calculus of Vector-Valued Functions, 11.7 – Motion in Space, and 11.8 – Length of CurvesĬhapter 12 – Functions of Several Variablesġ2.1 – Planes and Surfaces, 12.2 – Graphs and Level Curves, 12.4 – Partial Derivatives, 12.5 – The Chain Rule, 12.6 – Directional Derivatives and the Gradient, 12.7 – Tangent Planes and Linear Approximations, 12.8 – Maximum/Minimum Problems, 12.9 – Lagrange Multipliersġ3.1 – Double Integrals over Rectangular Regions, 13.2 – Double Integrals over General Regions, 13.3 – Double Integrals in Polar Coordinates, 13.4 – Triple Integrals, 13.5 – Triple Integrals in Cylindrical and Spherical Coordinatesġ4.1 – Vector Fields and Integrals, 14.2 – Line Integrals, 14.3 – Conservative FieldsĬhapter 14, Part II – Vector Calculus – Part IIġ4.4 – Green’s Theorem, 14.5 – Divergence and Curl, 14.6 – Surface Integrals, 14.7 – Stokes’ Theorem, 14.8 – Divergence Theorem The links below contain review material for an undergraduate-level course on multivariable calculus.


 0 kommentar(er)
0 kommentar(er)
